D. Depth and Diameter

Appendix D. Bullet Penetration Depth and Expanded Diameter

Discovering that penetration depth is more or less constant within the design velocity range allows us to establish trends with much less effort than might otherwise be the case. The author of the Terminal Ballistics paper at rathcoombe.net generated and accumulated a significant database of penetration depths and expanded bullet diameters in a variety of calibers and bullets. This data was used for developing relations for penetration depth and expanded bullet diameter. The expanded diameter is used to estimate the diameter of the wound channel in the algorithm described in Appendix A.

Examination of this data showed that penetration depth is not dependent on initial sectional density. Figure D-1 shows an example of penetration depth plotted against the initial sectional density for bonded bullets. We can see that there is no dependence on this parameter.

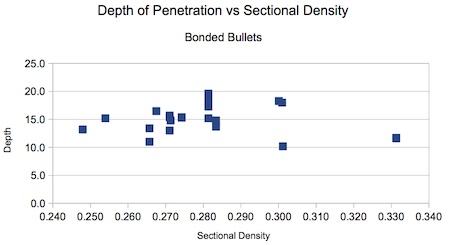
Figure D-1. In-Flight Sectional Density does not appear to be a parameter for penetration depth with expanding bullets.

Plotting against bullet weights for calibers gives a distinct, though still fuzzy, trend as shown in Figure D-2. Note that the red line is the trend for all 120-plus data points for all four bullet types. As one should expect, the blue line running below the red line indicates that cup & core designs do not penetrate as deeply as the other classes of bullet.

Figure D-2. Penetration Depth can be represented by a power function in the bullet weight.

The trend is good enough to allow the use of power functions for all four classes of bullet construction used in the tests. The resulting expressions are of the form

Turning to expanded diameter, we see that the trend is similar but that the cup and core bullets show more nearly an average degree of expansion. This is partly because the all-copper designs tend to not expand to as large a final diameter as the other bullet designs.

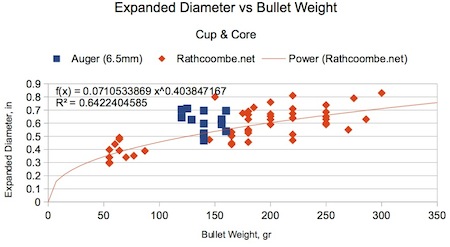
Figure D-3. Cup and Core bullet diameter is best modeled as a power function of the bullet’s weight.

The data also suggests that a power function is suitable for approximating the expanded bullet diameter as a function of weight:.

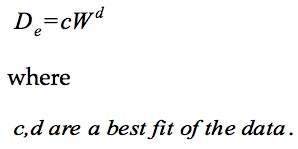
The penetration for a particular bullet weight and construction is then compared with the ribcage and thorax thicknesses to estimate the length of the probable wound channel inside the thorax. The wound channel diameter is assumed to be the same as the nominal diameter of the expanded bullet. These two numbers define the length and diameter of the ‘tube’ we use to represent the wound channel, and the resulting surface area is compared against the areas described in Appendix B using the methodology outlined in Appendix A.

# # # #